-

Existence of Traveling Waves for Ratio-dependent Predator-prey System with Nonlocal Diffusion
Issue:
Volume 8, Issue 5, October 2020
Pages:
236-246
Received:
18 February 2020
Accepted:
14 August 2020
Published:
25 August 2020
Abstract: In this paper, we study the traveling waves for the ratio-dependent predator-prey model with nonlocal diffusion, which is devoted to the existence and nonexistence of traveling wave solution. This model incorporates the ratio-dependent functional response into the Lotka-Volterra type system, and both species obey the logistic growth. Firstly, we construct a nice pair of upper and lower solutions when the wave speed is greater than the minimal wave speed. Then by applying Schauder's fixed point theorem with the help of suitable upper and lower solutions, we can obtain the existence of traveling waves when the wave speed is greater than the minimal wave speed. Moreover, in order to prove the limit behavior of the traveling waves at infinity, we construct a sequence that converges to the coexistence state. Finally, by using the comparison principle, we obtain the nonexistence of the traveling waves when the wave speed is greater than 0 and less than the minimal wave speed. The difficulty of this paper is to construct a suitable upper and lower solution, which is also the novelty of this paper. Under certain restricted condition, this paper concludes the existence and the nonexistence of the traveling waves for the ratio-dependent predator-prey model with nonlocal diffusion.
Abstract: In this paper, we study the traveling waves for the ratio-dependent predator-prey model with nonlocal diffusion, which is devoted to the existence and nonexistence of traveling wave solution. This model incorporates the ratio-dependent functional response into the Lotka-Volterra type system, and both species obey the logistic growth. Firstly, we co...
Show More
-

Mathematical Epidemiology Model Analysis on the Dynamics of COVID-19 Pandemic
Abayneh Fentie Bezabih,
Geremew Kenassa Edessa,
Purnachandra Rao Koya
Issue:
Volume 8, Issue 5, October 2020
Pages:
247-256
Received:
2 June 2020
Accepted:
28 June 2020
Published:
8 September 2020
Abstract: In the present work, Susceptible-Exposed-Infected-Recovered-Susceptible (SEIRS) mathematical model for COVID-19 Pandemic is formulated and analyzed. The positivity, boundedness, and existence of the solutions of the model are proved. The Disease-free equilibrium point and endemic equilibrium points are identified. Local Stability of disease-free Equilibrium point is checked with the help of Next generation matrix. Global stability of endemic equilibrium point is proved using the Concept of Liapunove function. The basic reproduction number for Novel Corona virus pandemic is computed as R0 = (αβΛ) ⁄ [(δ + μ) (β + δ + μ) (γ + δ + μ)] which depend on six different parameters. It is observed that if basic reproduction number is less than one, then number of cases decrease over time and eventually the disease dies out, and if the basic reproduction number is equals to one, then number of cases are stable. On the other hand, if the basic reproduction number is greater than one, then the number of cases increase over time gets worth. Sensitivity analysis of the basic reproduction number is done with respect to each parameter. It is observed that only some parameters Λ, α, β have high impact on the basic reproduction number. Consequently, with real data on the parameter it is helpful to predict the disease persistence or decline in the present situation. Lastly, numerical simulations are given using DEDiscover software to illustrate analytical results.
Abstract: In the present work, Susceptible-Exposed-Infected-Recovered-Susceptible (SEIRS) mathematical model for COVID-19 Pandemic is formulated and analyzed. The positivity, boundedness, and existence of the solutions of the model are proved. The Disease-free equilibrium point and endemic equilibrium points are identified. Local Stability of disease-free Eq...
Show More
-

Numerical Solution of Elastohydrodynamic Lubrication for Sliding/Rolling Bearing for Non-newtonian Lubricant
Samuel Macharia Karimi,
Mark Kimathi,
Mathew Ngugi Kinyanjui
Issue:
Volume 8, Issue 5, October 2020
Pages:
257-264
Received:
12 August 2020
Accepted:
3 September 2020
Published:
11 September 2020
Abstract: There is always a demand in the industry sector to increase the efficiency of machine components to reduce wear and tear. This paper presents the numerical solution to the study of Elastohydrodynamic lubrication point contact for sliding/rolling bearing where the viscosity of the lubricant is non-Newtonian. The assumption that a lubricant is Newtonian reduces validation of the model hence the Reynolds-Eyring model in this research will incorporate the non-Newtonian nature of the lubricant of the bearing. The mathematical model comprises of Reynold-Eyring equation, film thickness, load balance, lubricant viscosity and lubricant density equations together with their boundary conditions. The Reynolds-Eyring equation governing the flow is non-linear hence the finite difference method numerical technique is used to discretize it together with the other two dimensional equations. These equations are solved simultaneously and Matlab software is used simulate the results. The film thickness and pressure profiles with various loads and speeds are presented. The findings note that an increase in load lowers the pressure and film thickness while an increase in the speed results to a direct increase in pressure and film thickness. A pressure spike is also noted at the exit of the bearing.
Abstract: There is always a demand in the industry sector to increase the efficiency of machine components to reduce wear and tear. This paper presents the numerical solution to the study of Elastohydrodynamic lubrication point contact for sliding/rolling bearing where the viscosity of the lubricant is non-Newtonian. The assumption that a lubricant is Newton...
Show More
-

Approximate Numerical Solution of Singular Integrals and Singular Initial Value Problems
Md. Habibur Rahaman,
M. Kamrul Hasan,
Md. Ayub Ali,
Md. Shamsul Alam
Issue:
Volume 8, Issue 5, October 2020
Pages:
265-270
Received:
6 August 2020
Accepted:
27 August 2020
Published:
21 September 2020
Abstract: Numerical integration is one of the important branch of mathematics. Singular integrals arises in different applications in applied and engineering mathematics. The evaluation of singular integrals is one of the most challenging jobs. Earlier different techniques were developed for evaluating such integrals, but these were not straightforward. Recently various order straightforward formulae have been developed for evaluating such integrals but; all these integral formulae depend on Romberg technique for more accurate results. Based on these integral formulae, different order (up to fifth) implicit methods have been developed for solving singular initial value problems. These implicit methods give better results than those obtained by implicit Runge-Kutta methods but; the derivation of such higher order formulae are not so easy. In this article, a new third order straightforward integral formula has been proposed for evaluating singular integrals. This new formula is able to evaluate more efficiently than others existing formulae, moreover it has the independent ability to calculate very near accurate result to the exact value of the numerical integrals. Based on this new integral formula a new third order implicit method has been proposed for solving singular initial value problems. The new method provides significantly better results than other existing methods.
Abstract: Numerical integration is one of the important branch of mathematics. Singular integrals arises in different applications in applied and engineering mathematics. The evaluation of singular integrals is one of the most challenging jobs. Earlier different techniques were developed for evaluating such integrals, but these were not straightforward. Rece...
Show More
-

Control of Cauchy Problem for a Laplacian Operator
Issue:
Volume 8, Issue 5, October 2020
Pages:
271-277
Received:
18 May 2020
Accepted:
25 August 2020
Published:
21 September 2020
Abstract: In this paper we study the control of an ill-posed system relating to the Cauchy problem for an elliptical operator. The control of Cauchy systems for an elliptical operator has already been studied by many authors. But it still seems to be globally an open problem. Of all the studies that have been done on this problem, it is assumed that the set of admissible couple-state must be nonempty to make sense of the problem. This is the case of J. L. Lions in [6] who gave various examples of the admissible set to make a sense of the problem. O. Nakoulima in [9] uses the regularization-penalization method to approach the problem by a sequence of well-posed control problems, he obtains the convergence of the processus in a particular case of the admissible set. G. Mophou and O. Nakoulima in [10] do the same study and obtain the convergence of the processus when the interior of the admissible set is non empty. In this work, we give an approximate solution without an additional condition on the set of admissible couple-state.We propose a method which consists in associating with the singular control problem a "family" of controls of well posed problems. We propose as an alternative the stackelberg control which is a multiple-objective optimization approach proposed by H. Von Stackelberg in [12].
Abstract: In this paper we study the control of an ill-posed system relating to the Cauchy problem for an elliptical operator. The control of Cauchy systems for an elliptical operator has already been studied by many authors. But it still seems to be globally an open problem. Of all the studies that have been done on this problem, it is assumed that the set ...
Show More
-

Local Approximate Forward Attractors of Nonautonomous Dynamical Systems
Issue:
Volume 8, Issue 5, October 2020
Pages:
278-283
Received:
6 May 2020
Accepted:
24 July 2020
Published:
25 September 2020
Abstract: Pullback dynamics of nonautonomous dynamical systems has been considerably developed. However, it is still a tough job to study forward dynamics of nonautonomous dynamical systems, since forward attractors were only obtained in some particular cases. In the paper, under some reasonable conditions, it is shown that closing to a local pullback attractor, there is an approximate forward attractor. Specifically, let ϕ be a cocycle semiflow on a Banach space X with driving system θ on a base space P. Suppose that the base space P is compact and ϕ is uniformly asymptotically compact. Let A(∙) be a local pullback attractor with 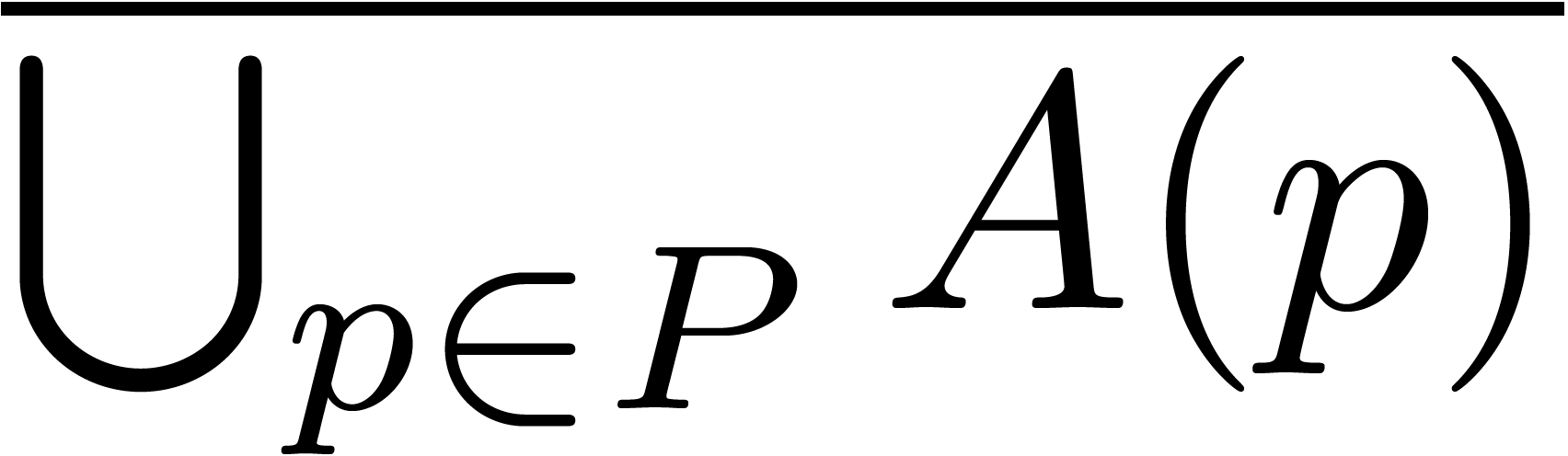 being compact. We prove that every ε-extended neighborhood Aε(∙) of A(∙) will forward attract every bounded set B(∙) that is pullback attracted by A(∙). We then call Aε(∙) an approximate forward attractor of ϕ.
Abstract: Pullback dynamics of nonautonomous dynamical systems has been considerably developed. However, it is still a tough job to study forward dynamics of nonautonomous dynamical systems, since forward attractors were only obtained in some particular cases. In the paper, under some reasonable conditions, it is shown that closing to a local pullback attrac...
Show More
being compact. We prove that every ε-extended neighborhood Aε(∙) of A(∙) will forward attract every bounded set B(∙) that is pullback attracted by A(∙). We then call Aε(∙) an approximate forward attractor of ϕ.
Abstract: Pullback dynamics of nonautonomous dynamical systems has been considerably developed. However, it is still a tough job to study forward dynamics of nonautonomous dynamical systems, since forward attractors were only obtained in some particular cases. In the paper, under some reasonable conditions, it is shown that closing to a local pullback attrac...
Show More
-

A Free Boundary Problem for a Leslie-Gower Predator-Prey Model in Higher Dimensions and Heterogeneous Environment
Shiwen Niu,
Hongmei Cheng
Issue:
Volume 8, Issue 5, October 2020
Pages:
284-292
Received:
14 September 2020
Accepted:
12 October 2020
Published:
26 October 2020
Abstract: This paper is mainly concerned with some free boundary problems for a modified Leslie-Gower predator-prey model in higher dimensional and heterogeneous environment. To keep it simple in this article, we assume that the environment and solutions are all radially symmetric. We consider the problem which be used to describe the spreading of an introduced predator species in higher dimensional and heterogeneous environment. We will assume that the prey is initially uniformly well disturbed. The prey undergoes the diffusion and growth in the entire space R^n. The predator is initially introduced in some localized location. We establish that a spreading-vanishing dichotomy is held for this model. We use the comparison principle. we will give the existence, uniqueness and some estimates of the solution to the problem. We study the asymptotic behavior of two species evolving. The free boundary represents the spreading front of the predator species. The boundary condition is described by classic Stefan-like condition. It is proved that the problem addressed is well posed, and that the predator species disperses to all domains in finite time. The long time behaviors of solution and criteria for spreading and vanishing of predator species are also provided. Furthermore, in the case that spreading of predator species happens, we deduce some rough estimates of the spreading speed.
Abstract: This paper is mainly concerned with some free boundary problems for a modified Leslie-Gower predator-prey model in higher dimensional and heterogeneous environment. To keep it simple in this article, we assume that the environment and solutions are all radially symmetric. We consider the problem which be used to describe the spreading of an introdu...
Show More






